Home » Converse of Base Angles Theorem
Category Archives: Converse of Base Angles Theorem
Two Column Geometry Proof problems
Two Column Geometry Proof Intersecting Lines example problem
Given: GA = RA, AH = AT
Prove: GT is congruent to RH
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Trapezoid example
Given: AD = 8, BC = 8, and BC is congruent to CD
Prove: AD is congruent to CD
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Circle example question
Given: 
is a diameter
Prove: (CD)2 = (AD)(DB)
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Triangle example problem
Given: 
Prove: 
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Triangle example #2
Given: 
with 
the bisector of 
Prove: BFDE is a rhombus
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Circle example question #2
Given: Circle with center O and diameter
Prove: 
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Kite example problem
Given: Kite ABCD with 
E, F, G, H are midpoints of 
Prove: EFGH is a rectangle
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Triangle example #3
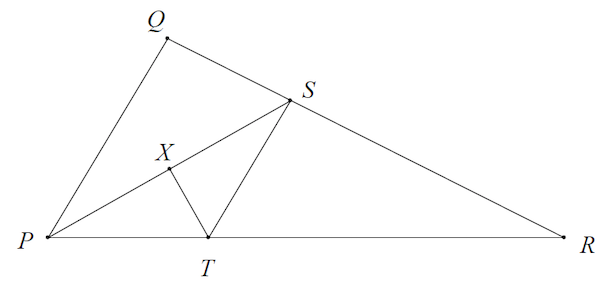
Given: 
bisects 
is a perpendicular bisector of
Prove: 
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Triangle example question #4
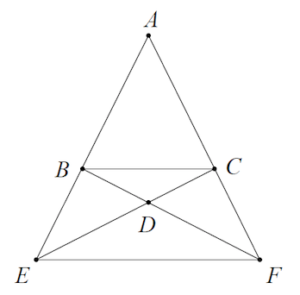
Given: is isosceles and 
Prove: 
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Two Column Geometry Proof Parallel Lines Transversal Triangle example problem
Given: 
bisects 
bisects 
Prove: 
Solution to this Two Column Geometric Proof practice problem is given in the video below!
Recent Comments