Home » Posts tagged 'calculus related rates example solutions'
Tag Archives: calculus related rates example solutions
Categories
- Absolute Value (2)
- Absolute Value Equations (1)
- Absolute Value Inequalities (1)
- ACT Math Practice Test (2)
- ACT Math Tips Tricks Strategies (25)
- Addition & Subtraction of Polynomials (2)
- Addition Property of Equality (2)
- Addition Tricks (1)
- Adjacent Angles (2)
- Albert Einstein's Puzzle (1)
- Algebra (3)
- Alternate Exterior Angles Theorem (1)
- Alternate Interior Angles Theorem (2)
- Alternating Series (1)
- Altitude of a Triangle (2)
- Angle Addition Postulate (1)
- Angle Angle Side Theorem (1)
- Angle Angle Similarity Postulate (1)
- Angle Bisector Theorem (2)
- Angle Side Angle Theorem (1)
- Angles (28)
- Angles Parallel Lines Transversals (1)
- Application of Differentiation (6)
- Application of Integration (8)
- Arc Addition Postulate (1)
- Arc Length of a Curve Integration (1)
- Area as a Function of Length (1)
- Area between Curves Integration (2)
- Arithmetic & Geometric Sequence (1)
- Asymptotes of Rational Functions (1)
- Atoms Molecules Ions (2)
- Avogadro Gas Law (2)
- Balancing Chemical Equations (2)
- Base Angles Theorem (1)
- Binary Ionic Compound Formulas (2)
- Binomial Distribution (4)
- Binomial Theorem (2)
- Bounded Sequence (1)
- Boyle Gas Law (2)
- Business Calculus (1)
- Calorimetry (1)
- CASTC Theorem (1)
- Central Angle (2)
- Centroid (1)
- Chain Rule of Derivatives (1)
- Charles Gas Law (2)
- Chemical Reactions in Aqueous Solutions (5)
- Chemistry Matter and Measurement (2)
- Circles (3)
- Circumcenter (1)
- Combined Gas Law (2)
- Combined Variation and Proportion (1)
- Combining Like Terms in Polynomials (1)
- Commutative Property of Multiplication (1)
- Comparison Test (1)
- Completing the Square (2)
- Complex and Imaginary Numbers (2)
- Composition of Functions (2)
- Compound Interest (1)
- Conditional Expectation (1)
- Conditional Probability (2)
- Confidence Intervals (1)
- Conic Sections (1)
- Consecutive Exterior Angles Theorem (1)
- Consecutive Interior Angles Theorem (2)
- Continuity (3)
- Continuous Random Variable (8)
- Converse of Base Angles Theorem (1)
- Corresponding Angles Theorem (1)
- Covariance (1)
- CPCTC Theorem (1)
- CSSTP Theorem (1)
- Cumulative Distribution (1)
- Curve Sketching with Differentiation (2)
- Cylindrical Coordinates (1)
- Cylindrical Shell Integration Method (2)
- Decimal and Rational Number Operations (1)
- Derivative of Exponential Functions (3)
- Derivative of Hyperbolic & Inverse Hyperbolic Functions (3)
- Derivative of Inverse Functions (3)
- Derivative of Inverse Trigonometric Functions (3)
- Derivative of Logarithmic Functions (3)
- Differential Equations (10)
- Differentials (1)
- Dilation (1)
- Direct Variation and Proportion (1)
- Discrete Mathematics and Combinatorics (4)
- Discrete Random Variable (7)
- Disk Integration Method (2)
- Distributive Property of Multiplication (1)
- Division Property of Equality (2)
- Division Tricks (1)
- Domain and Range of a Function (1)
- Double Integrals (4)
- Eigenvalues and Eigenvectors (1)
- Ellipse (1)
- Empirical and Molecular Formula (2)
- Enthalpy Change (2)
- Equilateral Triangles (1)
- Euler (1)
- Expected Value (5)
- Exponential Distribution (1)
- Exponential Equations (3)
- Exponents (3)
- Exterior Angle Theorem (1)
- Exterior Angles (1)
- Factoring Polynomials (3)
- Financial Math (6)
- First Law of Thermodynamics (2)
- Fourier Series (1)
- Fraction Multiplication Rule (1)
- Fractions (7)
- Fundamental Theorem of Calculus (2)
- Gas Pressure (2)
- Gases in Chemistry (7)
- GED Math (4)
- Geometric Distribution (1)
- Geometric Formula as a Function (1)
- Geometric Formula Proof with Integration (2)
- Geometric Series (1)
- Geometry (14)
- Graph of Trigonometric Functions (2)
- GRE Math (4)
- Greatest Common Factor (1)
- Harmonic Series (1)
- Hydrostatic Force & Work (3)
- Hyperbola (1)
- Hypergeometric Distribution (2)
- Hypotenuse Leg Theorem (1)
- Hypothesis Testing (1)
- Ideal Gas Law (2)
- Identity Property of Multiplication (1)
- Implicit Differentiation (7)
- Improper Integrals (7)
- Incenter (1)
- Increasing or Decreasing Sequence (1)
- Independent Events Probability (1)
- Infinite Sequences (8)
- Infinite Series (19)
- Infinite Series Derivative (1)
- Inscribed Angle (1)
- Integral Test (1)
- Integration by Partial Fraction Decomposition (6)
- Integration by Parts (6)
- Integration by Trigonometric Substitution (5)
- Integration of Exponential Functions by Substitution (5)
- Integration of Functions with Roots & Fractions (5)
- Integration of Hyperbolic & Inverse Hyperbolic Functions by Substitution (5)
- Integration of Inverse Trigonometric Functions by Substitution (5)
- Integration of Logarithmic Functions by Substitution (5)
- Integration of Trigonometric Functions by Substitution (5)
- Integration with Reduction Formula (1)
- Interior Angles (2)
- Inverse Functions (2)
- Inverse Trigonometric Functions (1)
- Inverse Variation and Proportion (1)
- Isosceles Triangles (1)
- Joint Distribution (1)
- Joint Moment Generating Functions (1)
- Joint Variation and Proportion (1)
- Kite (1)
- Lattice Multiplication (1)
- Law of Combining Volumes (2)
- Law of Cooling (1)
- Law of Cosines (1)
- Law of Sines (1)
- Laws of Chemical Combination (2)
- Least Common Multiple (1)
- Limit Comparison Test (1)
- Limit Definition of Derivative (3)
- Limiting Reactants (2)
- Limits (10)
- Line Segments (1)
- Linear Algebra (6)
- Linear Approximation (2)
- Linear Equations (3)
- Linear Functions (1)
- Linear Measure (1)
- Linear Pair Angles Theorem (2)
- Locus of Points (1)
- Logarithmic Differentiation (2)
- Logarithmic Equations (1)
- Logarithms (4)
- Maclaurin Series (1)
- Mass Percent Composition from Chemical Formulas (2)
- Math Puzzles (2)
- Math Tricks (7)
- Matrices (5)
- Mean Value Theorem for Integrals (1)
- Means-Extremes Property of Proportions (2)
- Median of a Triangle (1)
- Midsegment Theorem (1)
- Molar Mass (2)
- Molarity of Compounds and Ions (2)
- Mole and Avogadro's Number (2)
- Molecular and Formula Mass (2)
- Moment Generating Function (2)
- Multiple Integrals (2)
- Multiplication Property of Equality (2)
- Multiplication Tricks (1)
- Multivariable Calculus (5)
- Music Theory (3)
- Negative Binomial Distribution (1)
- Negative Exponents (1)
- NonLinear Equations (8)
- NonLinear Inequalities (2)
- Normal Approximation (1)
- Normal Distribution (3)
- Nth Term Test (1)
- Number Line (5)
- Optimization (4)
- Orthocenter (2)
- Oxidation and Reduction in Chemical Reactions (2)
- P Series (1)
- Parabola (3)
- Parametric Equations and Curves (2)
- Partial Derivatives (2)
- Partial Fraction Decomposition (1)
- Particle Motion (1)
- Pascal's Triangle (1)
- Percentage Probability (1)
- Perimeter as a Function of Area (1)
- Permutations and Combinations (1)
- Perpendicular Bisector Theorem (1)
- Physics (1)
- Piecewise Probability Distribution Functions (4)
- Plane (1)
- Poisson Distribution (2)
- Polar Coordinates (2)
- Polynomial Multiplication (1)
- Power Rule of Derivatives (5)
- Power Series (1)
- Precalculus (2)
- Precipitate Formation in Chemical Reactions (2)
- Principle of Mathematical Induction (1)
- Probability & Statistics (25)
- Probability of Outcomes (1)
- Product Rule of Derivatives (1)
- Projectile Motion (3)
- Puzzles (6)
- Quadratic Equations (3)
- Quadratic Formula (1)
- Quadratic Functions (2)
- Quadratic Inequalities (1)
- Quotient Rule of Derivatives (1)
- Radians Degrees Minutes Seconds (2)
- Radicals (1)
- Rational and Radical Expressions (3)
- Rational Equations (1)
- Rational Inequalities (1)
- Rational Numbers (2)
- Reactions of Acids and Bases (2)
- Reciprocal Rule of Division (1)
- Rectangles (1)
- Reference Angles (1)
- Reflection (2)
- Reflexive Property (1)
- Related Rates (3)
- Riemann Sum (20)
- Right Triangles (1)
- Rotation (2)
- Rotation of Axes (1)
- Rule of Product (1)
- Russian Peasant Multiplication (1)
- SAT Math Practice Test #1 (59)
- SAT Math Practice Test #2 (59)
- SAT Math Practice Test #3 (59)
- SAT Math Practice Test #4 (59)
- SAT Math Practice Test #5 (30)
- SAT Math Practice Test #6 (25)
- SAT Math Practice Test #7 (12)
- SAT Math Practice Test #8 (3)
- Scientific Notation (2)
- Segment Addition Postulate (1)
- Side Angle Side Theorem (1)
- Side Side Side Theorem (1)
- Sidelight Division (1)
- Significance Tests (1)
- Significant Figures (2)
- Similarity of Triangles (1)
- SOA Exam P (4)
- Solve for X (2)
- Specific Heat (1)
- Spherical Coordinates (1)
- Square Number (1)
- Squares (1)
- Standard Deviation (3)
- Stoichiometry Chemical Calculations (8)
- Substitution Property of Equality (2)
- Subtraction Property of Equality (2)
- Summation Formulas (2)
- Supplementary Angle Theorem (1)
- Surface Area Integration (2)
- Symmetric Property (2)
- Symmetry Tests (1)
- Synthetic and Long Division of Polynomials (1)
- System of NonLinear Equations (1)
- Systems of Linear Equations (1)
- T Distribution (1)
- Table Data (3)
- Table of Ordered Pairs (1)
- Taylor Series (1)
- Telescoping Series (1)
- Theoretical & Proofs (5)
- Thermochemistry (3)
- Titrations in Chemical Reactions (2)
- Transformation (5)
- Transitive Property (2)
- Translation (1)
- Trapezoid (1)
- Tree Diagram Probability (1)
- Triangles (22)
- Trigonometric Angle Sum Difference Multiple Half-Angle Formulas (3)
- Trigonometric Equations (4)
- Trigonometric Form of Complex Numbers (1)
- Trigonometric Functions (4)
- Trigonometric Identity Proofs (1)
- Trigonometry (19)
- Triple Integrals (1)
- Truth Tables (1)
- Two Column Proofs (2)
- Two Way Table (1)
- Uniform Distribution (1)
- Unit Circle (5)
- Unit Conversions (2)
- Variance (2)
- Variation and Proportion (4)
- Vectors (1)
- Vectors in the Plane (2)
- Venn Diagram (1)
- Vertex Form of a Parabola (1)
- Vertical Angles Theorem (2)
- Volume of the Solid of Revolution (2)
- Washer Integration Method (2)
- Whole Number Operations (3)
- World's HARDEST Easy Geometry problems (1)
- Wronskian (1)
- Yield of Chemical Reactions (2)
Related Rates calculus problems
April 5, 2019 / Leave a comment
Sphere Related Rates example problem
The radius of a sphere is r when the time is t seconds. Find the radius when the rate of change of the surface area and the rate of change of the radius are equal.
Solution to this Calculus Related Rates practice problem is given in the video below!
Balloon Related Rates example question
Gas is escaping from a spherical balloon at the rate of 4 cubic feet per minute. How fast is the surface area of the balloon shrinking when the radius of the balloon is 4 feet?
Solution to this Calculus Related Rates practice problem is given in the video below!
Approaching Cars Related Rates example
A car is traveling at 50 miles per hour due south at a point ![]() mile north of an intersection. A police car is traveling at 40 miles per hour due west at a point
mile north of an intersection. A police car is traveling at 40 miles per hour due west at a point ![]() mile east of the same intersection. At that instant, the radar in the police car measures the rate at which the distance between the two cars is changing. What does the radar gun register?
mile east of the same intersection. At that instant, the radar in the police car measures the rate at which the distance between the two cars is changing. What does the radar gun register?
Solution to this Calculus Related Rates practice problem is given in the video below!
Sliding Ladder Related Rates example problem
A 10-foot ladder leans against the side of the building. If the top of the ladder begins to slide down the wall at the rate of 2 feet per second, how fast is the bottom of the ladder sliding away from the wall when the top of the ladder is 8 feet off the ground?
Solution to this Calculus Related Rates practice problem is given in the video below!
Oil Spill Related Rates example question
An oil tanker has an accident and oil pours out at the rate of 150 gallons per minute. Suppose that the oil spreads onto the water in a circle at a thickness of ![]() inches. Given that 1 cubic inch equals 7.5 gallons, determine the rate at which the radius of the spill is increasing when the radius reaches 500 feet.
inches. Given that 1 cubic inch equals 7.5 gallons, determine the rate at which the radius of the spill is increasing when the radius reaches 500 feet.
Solution to this Calculus Related Rates practice problem is given in the video below!
Trigonometry Related Rates example
A spectator at an air show is trying to follow the flight of a jet. The jet follows a straight path in front of the observer at 540 miles per hour. At its closest approach, the jet passes 600 feet in front of the person. Find the maximum rate of change of the angle between the spectator’s line of sight and a line perpendicular to the flight path, as the jet flies by.
Solution to this Calculus Related Rates practice problem is given in the video below!
Complex Trigonometry Related Rates example problem
Two buildings, of height 20 feet and 40 feet, are 60 feet apart. Suppose that the intensity of light at a point between the buildings is proportional to the angle ![]() in the figure. If a person is moving from right to left at 4 feet per second, at what rate is this angle
in the figure. If a person is moving from right to left at 4 feet per second, at what rate is this angle ![]() changing when the person is exactly halfway between the two buildings?
changing when the person is exactly halfway between the two buildings?
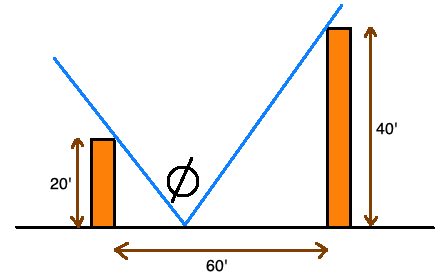
Solution to this Calculus Related Rates practice problem is given in the video below!
Cone Related Rates example question
Water is running out of a conical funnel at the rate of 1 cubic inch per second. If the radius of the base of the funnel is 4 inches and the height is 8 inches, find the rate at which the water level is dropping when it is 2 inches from the top.
Solution to this Calculus Related Rates practice problem is given in the video below!
Pulley example Related Rates example
A weight W is attached to a rope 50-foot-long that passes over a pulley at a point P, 20 feet above the ground. The other end of the rope is attached to a truck at a point A, 2 feet above the ground. If the truck moves away at the rate of 9 feet per second, how fast is the weight rising when it is 6 feet above the ground?
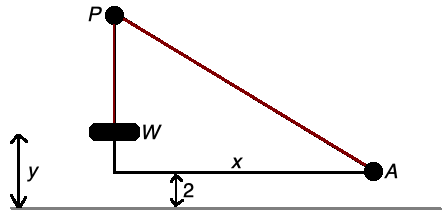
Solution to this Calculus Related Rates practice problem is given in the video below!
Shadow Related Rates example problem
Suppose a 6-ft-tall person is x feet away from an 18-ft-tall lamppost. If the person is moving away from the lamppost at a rate of 2 feet per second, at what rate is the length of the shadow changing?
Solution to this Calculus Related Rates practice problem is given in the video below!
Kite Related Rates example question
A boy is flying a kite at a height of 150 feet. If the kite moves horizontally away from the boy at 20 feet per second, how fast is the string being paid out when the kite is 250 feet from him?
Solution to this Calculus Related Rates practice problem is given in the video below!
Curve Related Rates example
If a point moves along the curve ![]() ,
,
at what point is the y-coordinate changing twice as fast as the x-coordinate?
Solution to this Calculus Related Rates practice problem is given in the video below!
Boat Related Rates example problem
A barge, whose deck is 10 feet below the level of the dock, is being drawn in by means of a cable attached to the deck and passing through a ring on the dock. When the barge is 24 feet away and approaching the dock at ![]() feet per second, how fast is the cable being pulled in? (Neglect any sag in the cable.)
feet per second, how fast is the cable being pulled in? (Neglect any sag in the cable.)
Solution to this Calculus Related Rates practice problem is given in the video below!
HARD Related Rates Clock example question
The minute and hour hands of a clock are 15 cm and 10 cm long, respectively. Find the rates at which the angle and distance between the tips of these hands of a clock are changing when the time on the clock shows 12:30 p.m.
Solution to this Calculus Related Rates practice problem is given in the video below!
Recent Posts
- Angular Speed and Linear Speed problems March 13, 2024
- Converting Repeating Decimals to Fractions problems February 29, 2024
- Geometry Puzzles and Brain Teasers December 18, 2023
- VERIFYING Whether Given Two Functions are Inverses December 15, 2023
- Trigonometric Functions WORD problems December 11, 2023
- Null Hypothesis Testing statistics problems February 9, 2021
- Variance problems February 9, 2021
- Confidence Interval statistics problems December 9, 2020
- Greatest Common Factor and Least Common Multiple problems December 3, 2020
- Translation Geometry problems December 1, 2020
Archives
- March 2024 (1)
- February 2024 (1)
- December 2023 (3)
- February 2021 (2)
- December 2020 (4)
- November 2020 (4)
- October 2020 (12)
- September 2020 (10)
- August 2020 (2)
- July 2020 (9)
- June 2020 (2)
- May 2020 (22)
- April 2020 (5)
- November 2019 (1)
- May 2019 (409)
- April 2019 (62)
- March 2019 (63)
| S | M | T | W | T | F | S |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | |
| 7 | 8 | 9 | 10 | 11 | 12 | 13 |
| 14 | 15 | 16 | 17 | 18 | 19 | 20 |
| 21 | 22 | 23 | 24 | 25 | 26 | 27 |
| 28 | 29 | 30 | 31 | |||
Recent Comments